
Finally, a system can have no solutions if there are no points that satisfy the equations in the system. This is represented by two overlapping lines in two dimensions or two intersecting planes in three dimensions. A system has infinitely many solutions if there are infinitely many points that satisfy all of the equations in the system. This is represented by two intersecting lines in two dimensions or three intersecting planes in three dimensions. A system that has one solution will have only one point where the equations agree. There are three possible solutions that a system of equations could have. With a variable eliminated, the remaining equation or equations can then be more easily solved. The elimination method is the method of solving a system of equations by adding or subtracting the equations in the system in order to eliminate one or more variables. There are many ways to solve systems of equations, or find where the equations agree. Ī system of equations is a set of two or more equations in two or more variables.
System of equations solver elimination how to#
This will show how to solve by eliminating a system of three equations in three variables. Here is an example of how to solve systems by elimination. In this lesson, only the case where solutions exist will be shown, but it should be noted that the system of equations elimination method can result in any of the possible solution types. Here is an image depicting this solution type in two dimensions in Figure 3:įig. This solution type is represented by two non-intersecting lines in two dimensions or two non-intersecting lines or planes in three dimensions. No solutions: The system of equations has no solutions that satisfy all of the equations.2: System of Equations: Infinite Solutions Here is an image in Figure 2 showing this solution type in two dimensions:įig.
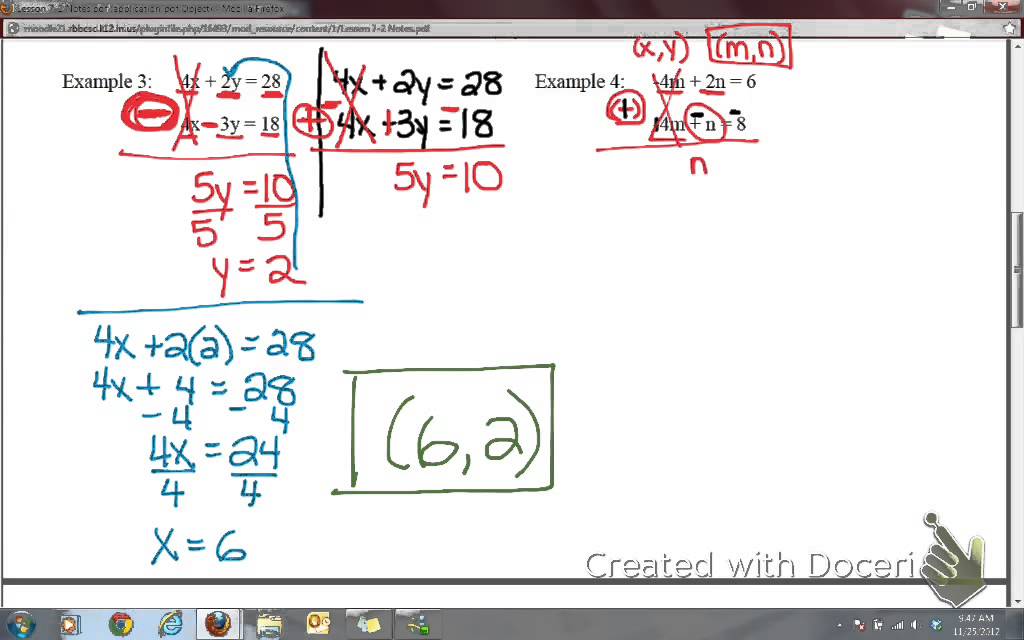
This solution type is represented by two overlapping lines in two dimensions or two intersecting or overlapping planes in three dimensions. Infinite solutions: The system of equations has infinitely many solutions or infinitely many points satisfy the system of equations.Here is an image in Figure 1 showing this solution type in two dimensions:įig. This solution type is represented as two intersecting lines in two dimensions or three intersecting planes in three dimensions. One solution: The system of equations has a single solution that satisfies all of the equations in the system.A system of equations in which elimination is used is typically called a system of elimination.įurthermore, systems of equations have one of three possible solution types: This is because the elimination method involves eliminating a variable.
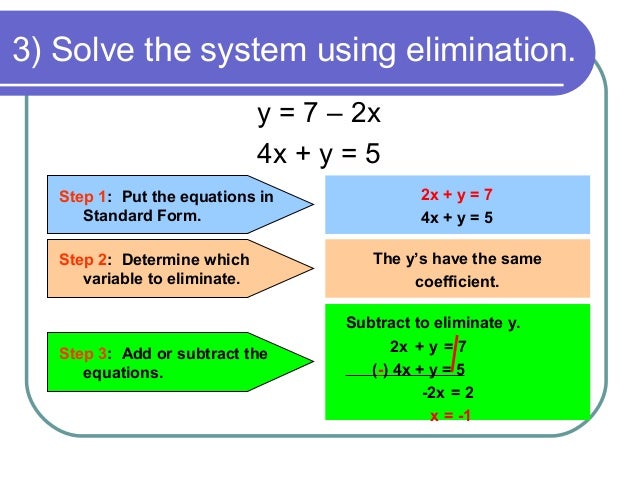
The elimination method is typically used when the system of equations has two or more equations in two or more variables. The remaining variables can then be found. This method results in a single equation in one variable which can then be solved. The elimination method for a system of equations involves adding or subtracting the equations in the system in order to eliminate one or more variables. One method of solving a system of equations is the elimination method:

A system of equations is a set of two or more equations involving two or more variables.


 0 kommentar(er)
0 kommentar(er)
